Commutativity of Prime Ring with Orthogonal Symmetric Biderivations
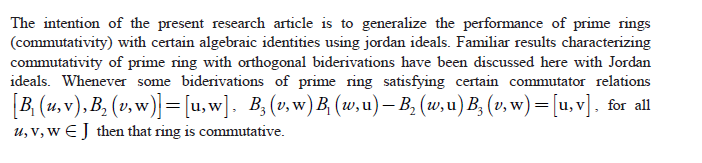
Abstract:
Author(s):
DOI:
Keywords:
References:
Argac, N., Nakajima, A., Albas, E. (2004). On orthogonal generalized derivations of semiprime Rings. Turk.j.Math. 28, 185–194.
Ashraf, M., Jamal, M. R. (2010). Orthogonal derivations in gamma-rings. Advances in Algebra. 3(1), 1–6.
Bell, H. E., Daif, M. N. (1995). On derivations and commutativity in prime rings. Acta Mathematica Hungarica. 66, 337–343. https://doi.org/10.1007/BF01876049
Bresar, M. (1993). Centralizing mappings and derivations in prime rings. J. Algebra. 156, 385–394. https://doi.org/10.1006/jabr.1993.1080
Bresar. M., Vukman, J. (1989). Orthogonal derivation and extension theorem of posner. Radovi Mathematicki. 5,237–246.
Bresar. M., Vukman, J. (1988). Jordan derivations of prime rings. Bull.Aust.Math.Soc. 37, 321–324. https://doi.org/10.1017/S0004972700026927.
Cusack, J. M. (1975). Jordan derivations in rings. Proc. Am.Math.Soc. 53(2), 321–324.
Daif, M. N. (1998). Commutativity result for semiprime rings with derivations. Int.J.Math.Sci. 21(3), 471–474.
Herstein, I. N. (1976). Rings with Involution. Uni.Chicago press. Chicago.
Oukhtite, L. (2011). Posner’s second theorem for Jordan ideals in rings with involution. Expo. Math. 29(4), 415– 419. https://doi.org/10.1016/j.exmath.2011.07.002
Oukhitite. L., Mamouni. M., Beddani, C. (2014). Derivations and Jordan ideals in prime Rings. Journal of Taibah University for Science. 8, 364–369. https://doi.org/10.1016/j.jtusci.2014.04.004
Posner, E. C. (1957). Derivations in prime rings. Proc. Am.Math.Soc. 8, 1093–1100.
Reddy. C. J. S., Reddy, B. R. (2016). Orthogonal Symmetric bi-derivations in semiprime rings. International journal of Mathematics and statistics studies. 4(1),22–29.
Vukman, J. (1989). Symmetric biderivations on prime and Semiprime rings. Aeq.Math. 38, 245–254. https://doi.org/10.1007/BF01840009
Vukman, J. (1990). Two results concerning symmetric biderivations on prime rings. Aeq.Math. 40, 181–189. https://doi.org/10.1007/BF02112294




