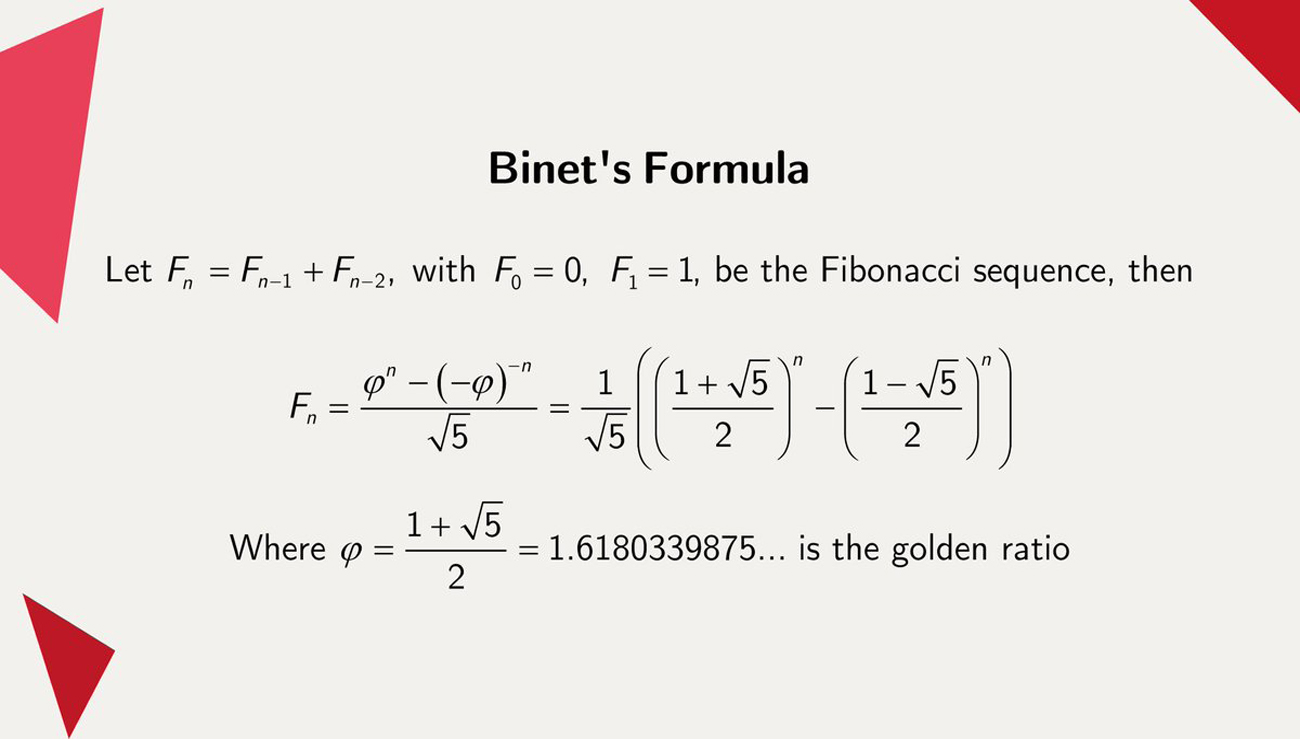
Binet – Type Formula For The Sequence of Tetranacci Numbers by Alternate Methods

September 1, 2017

Binet – Type Formula For The Sequence of Tetranacci Numbers by Alternate Methods

Abstract:

The sequence {T_n} of Tetranacci numbers is defined by recurrence relation T_n= T_n-1 + T_n-2 + T_n-3 + T_n-4; n≥4 with initial condition T₀=T₁=T₂=0 and T₃=1. In this Paper, we obtain the explicit formulla-Binet-type formula for T_n by two different methods. We use the concept of Eigen decomposition as well as of generating functions to obtain the result.

Author(s):

Gautam S. Hathiwala, C.K. Pithawala College of Enigneering & Technology, Surat.
Devbhadra V. Shah, Department of Mathematics, Veer Narmad South Gujarat University, Surat.

DOI:

https://doi.org/10.15415/mjis.2017.61004

Keywords:

Binet formula, Fibonacci sequence, Tetranacci sequence

References:

Arfken, G.: Diagonalization of Matrices, Mathematical Methods for Physicists, 3rd ed. Orlando, FL: Academic Press, 1985, 217–229.

Dresden G.P.B., Du Z.: A Simplified Binet Formula for Generalized Fibonacci Numbers, Journal of Integer Functions, Vol. 17, article 14.4.7, 2014.

Eigen decomposition of a matrix: http://mathworld.wolfram.com/EigenDecompositionTheorem.html

Hathiwala G. S., Shah D. V.: Golden proportions for the generalized Tetranacci numbers, International Research Journal of Mathematics, Engineering and IT, Vol. 3, Issue 4, April 2016, 90–101.

Hathiwala G. S., Shah D. V.: “Periodicity of Tetranacci Numbers Modulo ”, The Journal of the Indian Academy of Mathematics, Vol. 38, Issue 2, 2016, pp.155–165, ISSN: 0970 – 5120.

Lee G. Y., Lee G. S., Kim J. S., Shin H. K.: The Binet Formula and Representations of Generalized Fibonacci Numbers, Fibonacci Quarterly, Vol. 39, No. 2, May 2001, 158–164.

Mehta D.A.: Ph.D. thesis entitled “Properties of the Sequences of Tribonacci Numbers and Generalized Cut–off Numbers”, Veer Narmad South Gujarat University, Surat, India, Oct. 2009.

Raab J.A.: A Generalization of the Connection between the Fibonacci Sequence and Pascal’s Triangle, The Fibonacci Quarterly, Vol. 1, No. 3, Oct. 1963,21–32.

Singh B., Bhatnagar S., Sikhwal O.: Fibonacci– Like Sequence, InternationalJournal of Advanced Mathematical Sciences, Vol. 1, No. 3, 2013, 145–151.

Waddill M.E.: Some Properties of Tetranacci Numbers modulo, The Fibonacci Quarterly, Vol. 30, No. 3, Aug. 1992, 232–238.

Waddill M.E.: The Tetranacci Sequence and Generalizations, The Fibonacci Quarterly, Vol. 30, No. 1, Feb. 1992, 9–20.

Wall D. D.: Fibonacci Series Modulo, American Math. Monthly, Vol. 67, 1960,525–532.

Chitkara University Publications