Continuous Models of Tumor Induced Angiogenesis and Anti-Angiogenesis Strategy
Abstract:
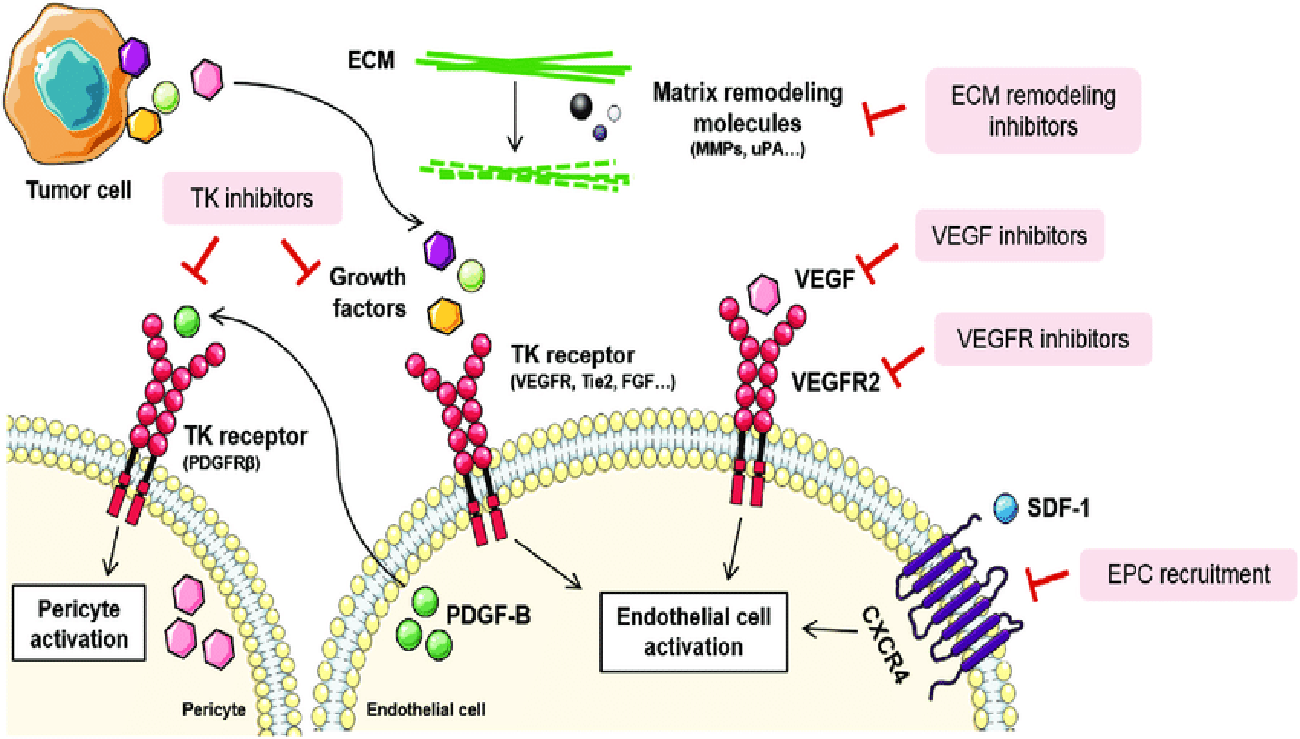
Continuous models of tumor-induced angiogenesis have been discussed and numerical simulations of one model have been carried out and the figures corresponding to various types of behaviors of the biological event have been shown. Actual dynamics of the biological process can be visualized with the help of figures generated. We also discussed the antiangiogenesis strategy by identifying factors responsible for angiogenesis and targeting such factors to control the process. The prevalent drugs along with their mathematical parameters have been discussed.
Author(s):
DOI:
Keywords:
References
Anderson, A.R.A. and Chaplain, M.A.J., (1998) Continuous and Discrete Mathematical Models of Tumor-induced Angiogenesis, Bull. Math. Biol., 60, 857-900. http://dx.doi.org/10.1006/bulm.1998.0042
Bahleda, R., Felip, E., Herbst, R., Hanna, N., Laurie, S., Shepherd, F., Armand, J.P., Sweeney, C., Calvo, E. and Soria, J.C., (2008) Phase I/II study of BMS-690514, an ErbB-VEGFR Inhibitor: Safety Pharmacodynamic Effects and Early Clinical Activity in Patients with Non-Small Cell Lung Carcinoma(NSCLS), Report, Bristol-Myers Squibb.
Balding, D. and McElwain, D.L.S., (1985) A Mathematical Model of Tumour-Induced Capillary Growth, J. Theor. Biol., 114, 53-73. http://dx.doi.org/ 10.1016/S0022-193(85)80255-1
Britten, C.D., Rosen, L.S., Kabbinavar, F., Rosen, P., Mulay, M., Hernandez, L., Brown, J., Bello, C., Kelsey S.M., Scigalla, P. (2002) Phase I trial of SU6668, a Small Molecule Receptor Tyrosine Kinase Inhibitor, Given Twice Daily in Patients with Advanced Cancers, Americal Society of Clinical Oncology Annual Meeting.
Bryne, H.M. and Chaplain, M.A.J., (1995) Mathematical Models for Tumour Angiogenesis: Numerical Simulations and Nonlinear Wave Solutions, Bulletin of Mathematical Biology, 57(3) 461-486. http://dx.doi.org/10.1016/S0092-8240(05)81778-1
Chaplain, M.A.J. and Stuart, A.M., (1991) A Mathematical Model for the Diffusion of Tumour Angiogenesis Factor into the Surrounding Host Tissue, IMA Journal of Mathematics Applied in Medicine and Biology, 8, 191-220.
Cristini, V., Lowengrub, J. and Nie, Q., (2003) Nonlinear Simulation of Tumor Growth, J. Math. Biol., 46, 191-224. http://dx.doi.org/10.1007/s00285-002-0174-6
Eddy, H.A. and Casarett, G.W., (1973) Development of the Vascular System in the Hamster Malignant Neurolemmoma, Microvasc. Res., 6, 63-82. ttp://dx.doi.org/10.1016/0026-2862(73)90007-1
Edelstein, L., (1982) The Propagation of Fungal Colonies: A Model for Tissue Growth, J. Theor. Biol., 98, 679-701. http://dx.doi.org/10.1016/0022-5193(82)90146-1
Folkman, J., (1976) The Vascularization of Tumours, Science Am., 234, 58-73. http://dx.doi.org/10.1038/scientificamerican0576-58
Gerisch, A. and Chaplain, M.A.J., (2008) Mathematical Modelling of Cancer Cell invasion of Tissue: Local and Non-Local Models and the Effect of Adhesion, J. of Theor. Biol., 250, 684-704. http://dx.doi.org/10.1016/j.jtbi.2007.10.026
Klenke, F.R. and Abdollahi, A., (2007) Tyrosine kinase inhibitor SU6668 represses chondrosarcoma growth via antiangiogenesis in vivo, BMC Cancer, 7. http://dx.doi.org/10.1186/1471-2407-7-49
Morabito, A., Piccirillo, M.C., Falasconi, F., DE Feo, G., Del Guidice, A., Bryce, J., Di Mai, E., Normanno, N., Perrono, F., (2009) Vandetanib(ZD6474), a Dual Inhibitor of Vascular
Endothelial Growth Factor Receptor(VEGFR) and Epidermal Growth Factor RAeceptor (EGFR), Oncologist,14, 378-390. http://dx.doi.org/10.1634/theoncologist.2008-0261
Paweletz, N. and Knierim, M., (1989) Tumour-Related Angiogenesis, Critical Reviews in Oncology/ Hematology, 9, 197-242.
Roose, T., Chapman, S. J. and Maini, P.K., (2008) Mathematical Models of Avascular Tumor Growth, SIAM Review, 49(2), 179-208. http://dx.doi.org/10.1137/S0036144504446291
Sachs, R.K., Hlatky, L.R. and Hahnfeldt, P., (2001) Simple ODE models of Tumor Growth and Anti-Angiogenic or Radiation Treatment, Mathematical and Computing Modelling, 33, 1297-1305. http://dx.doi.org/10.1016/S0895-7177(00)00316-2
Sherratt , J.A. and Chaplain, M.A.J., (2001) A New Mathematical Model for Avascular Tumour Growth, J. Math. Biol., 43, 291-312. http://dx.doi.org/10.1007/s002850100088
Stokes, C.L. and Lauffenburger, D.A., (1991) Analysis of the roles of microvessel endothelial cell random motility and chemotaxis in Angiogenesis, J. Theor. Biol., 152, 377-403.
http://dx.doi.org/10.1016/S0022-5193(05)80201-2
Swanson, K.R., Bridge, C., Murray, J.D., Alvord Jr, E.C., (2003) Virtual and Real brain Tumors: Using Mathematical Modeling to Quantify Glioma growth and Invasion, J. Neurological Sciences, 216, 1-10. http://dx.doi.org/10.1016/j.jns.2003.06.001
Travasso, Rui D.M., Poire, Castro, M., Rodrguez-Manzaneque, J.C. and Hernandez-Machado, A., (2011) Tumor Angiogenesis and Vascular Patterning: A Mathematical Model, PLoS ONE, 6 Wikipedia. http://dx.doi.org/10.1371/journal.pone.0019989




