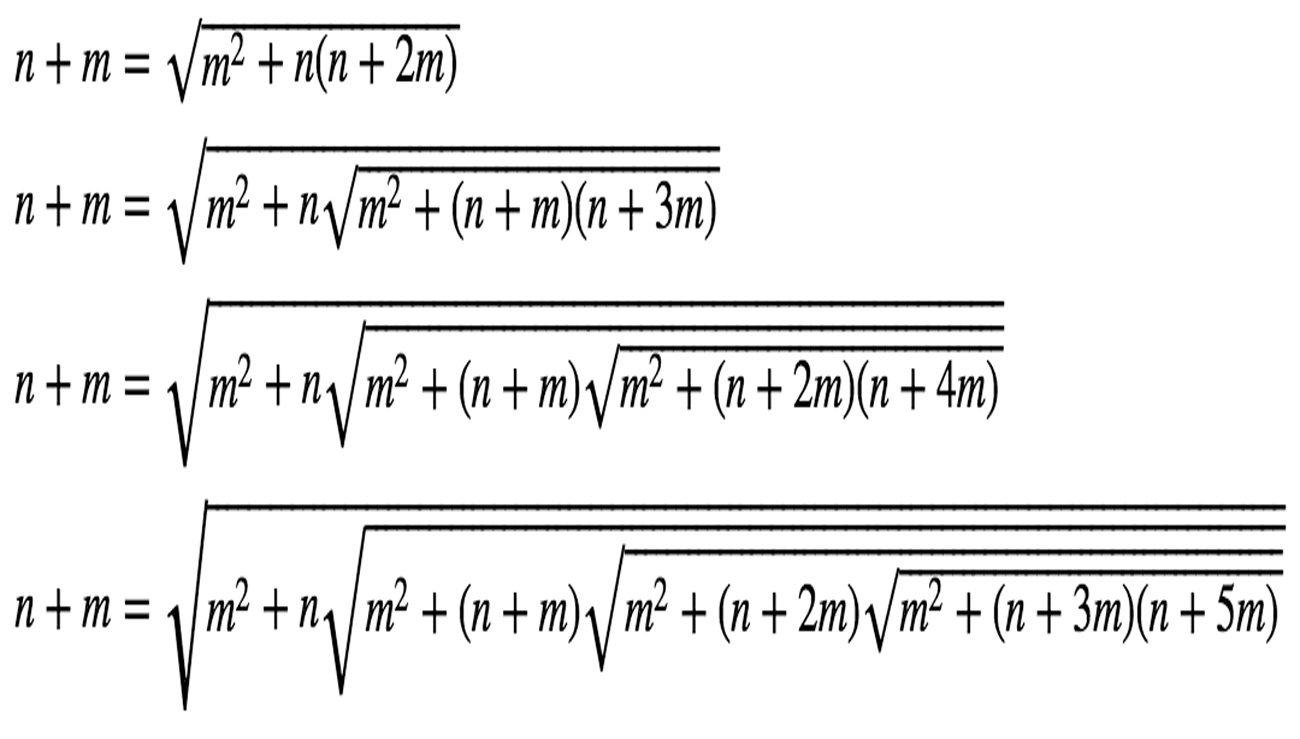
Infinite Nested Radicals – A Way to Express All Quantities Rational, Irrational Transcendental By a Single Integer Two
Abstract:
This paper proves that all mathematical quantities including fractions, roots or roots of root, transcendental quantities can be expressed by continued nested radicals using one and only one integer 2. A radical is denoted by a square root sign and nested radicals are progressive roots of radicals. Number of terms in the nested radicals can be finite or infinite. Real mathematical quantity or its reciprocal is first written as cosine of an angle which is expanded using cosine angle doubling identity into nested radicals finite or infinite depending upon the magnitude of quantity. The finite nested radicals has a fixed sequence of positive and negative terms whereas infinite nested radicals also has a sequenceof positive and negative terms but the sequence continues infinitely. How a single integer 2 can express all real quantities, depends upon its recursive relation which is unique for a quantity. Admittedly, there are innumerable mathematical quantities and in the same way, there are innumerable recursive relations distinguished by combination of positive and negative signs under the radicals. This representation of mathematical quantities is not same as representation by binary system where integer two has powers 0, 1, 2, 3…so on but in nested radicals, powers are roots of roots.
Author(s):
DOI:
https://doi.org/10.15415/mjis.2020.91001
Keywords:
Infinite Nested Radicals, Recursive Relation, Cosine Angle Doubling Identity, Integer Two, Mathematical Quantity, Modulus
References:
Herschfeld, A.: On Infinite Radicals. The American Mathematical Monthly 42(7), 419–429, (1935). https://doi.org/10.1080/00029890.1935.11987745 DOI: https://doi.org/10.1080/00029890.1935.11987745
Landau, S.: Simplification of Nested Radicals. SIAM Journal on Computing 21(1), 85–110 (1992). https://doi.org/10.1137/022100
Landau, S.: Simplification of Nested Radicals. 30th Annual Symposium on Foundations of Computer Science, Research Triangle Park, NC, USA, 1989, 314-319, https://doi.org/10.1109/SFCS.1989.63496 DOI: https://doi.org/10.1109/SFCS.1989.63496
Rick, K.: π to Thousands of Digits from Vieta’s Formula. Mathematics Magazine 81(3), 201–207, (2008).
Weisstein, Eric., Nested Radical. From MathWorld–A Wolfram Web Resource. https://mathworld.wolfram.com/NestedRadical.html
Zimmerman, S. and Ho, C.: On Infinitely Nested Radicals. Mathematics Magazine 81(1), 3–15, (2008).




